√99以上 one-to-one function example problems with solutions pdf 261757-One to one function example problems with solutions pdf

11 OneDimensional Functions 111 Solved Problem Problem 1 Consider the analytic function f R !R f(x) = 4x(1 x) (i) The xed points of the function fare the solutions of the equation f(x) = x Find the xed points (ii) The critical points of fare the solutions of the equation df(x)=dx= 0 Find the critical points of fSolution We have rw=(2xy;x), so (rw)( 1;1)=( 1;In this video I want to introduce you to some terminology that will be useful in our discussion of functions and invertibility and this is in general terminology that you'll probably see in your mathematical careers so let's say I have a function f and it is a mapping from the set X to the set Y and we've drawn this diagram many times but it never hurts to draw it again so that is my set X or

Solved 1 Show That Y X Is Not A One To One Function 2 Chegg Com

One to one function example problems with solutions pdf

One to one function example problems with solutions pdf-Sample Exponential and Logarithm Problems 1 Exponential Problems Example 11 Solve 1 6 3x 2 = 36x1 Solution Note that 1 6 = 6 1 and 36 = 62 Therefore the equation can be written (6 1) 3x 2 = (62)x1 Using the power of a power property of exponential functions, we can multiply the exponents 63x2 = 62x2 But we know the exponential function 6x is onetoone Therefore1) As the directional derivative in the direction !v at ( 1;1)is (rw)( 1;1) !v, which is maximized when !v =(rw)( 1;1)=( 1;1) Question #24 Let z(x;y)=f(x y)=y(where fis differentiable and y6=0 ), show that the identity zy@z @x y@z @y =0 Solution We have @z @y = f0(x y)( 1) y f(x y) y2;

Www Math Uh Edu Jiwenhe Math1432 Lectures Lecture01 Handout Pdf

406 CHaptER 4 Inverse Exponential and Logarithmic Functions OnetoOne Functions Suppose we define the following function F F = 512, 22, 11, 12, 10, 02, 11, 32, 12, 526 (We have defined F so that each second component is used only once) We can form another set of ordered pairs from F by interchanging the x and yvalues of each pair in FWe call this set GThere are 6 trigonometric functions The sinh and cosh functions are the primary ones;Example 31 The collection f(a;b) R a;b 2Qgis a basis for a topology on R Exercise 32 Show that collection of balls (with rational radii) in a metric space forms a basis Example 33 (Arithmetic Progression Basis) Let Xbe the set of positive integers and consider the collection B of all arithmetic progressions of positive integers

Determine where the function \(h\left( z \right) = 6 40{z^3} 5{z^4} 4{z^5}\) is increasing and decreasingTherefore, the solution to the problem ln(3x11)4 = is x ≈ Example – Solve 6 6 logxlog(x5)2 = This problem contains terms without logarithms This problem can be simplified by using Property 3 which changes the addition of logarithms to multiplicationKgk1 = Z 1 0 j2xjdx = 1;

Here we have the solutions to all the problemsin the second edition of Elements of Information Theory First a word about how the problems and solutions were generated The problems arose over the many years the authors taught this course At first the homework problems and exam problems were generated each week After a few years ofNote that the function is periodic of period 2 Solution Since f(t)e st e st;we have R 1 0 f(t)e stdt R 1 0 e stdtBut the integral on the right is convergent for s>0 so that the integral on the left is convergent as well That is, Lf(t) exists for s>0 The function of the above example belongs to a class of functions that we de ne nextPractice Problems Proofs and Counterexamples involving Functions Solutions The following problems serve two goals (1) to practice proof writing skills in the context of abstract function properties;

Solved 1 Show That Y X Is Not A One To One Function 2 Chegg Com

One To One Function Explanation Examples

 · Here is a set of practice problems to accompany the Differentiation Formulas section of the Derivatives chapter of the notes for Paul Dawkins Calculus I course at Lamar University Solution;Y(a) = y(b) = 0 are polygonal lines with y0 = §1 VThe Brachistochrone problem This is considered the oldestEXAMPLE PROBLEMS AND SOLUTIONS A31 Simplify the block diagram shown in Figure 342 Solution First, move the branch point of the path involving HI outside the loop involving H,, as shown in Figure 343(a) Then eliminating two loops results in Figure 343(b) Combining two blocks into one gives Figure 333(c) A32

List Of Unsolved Problems In Mathematics Wikipedia

Your Guide To The Python Print Function Real Python

Function #2 on the right side is the one to one function In a one to one function, every element in the range corresponds with one and only one element in the domain So, #1 is not one to one because the range element5 goes with 2 different values in the domain (4 and 11)F f(x 1) = f(x 2) = h Determining Whether a Function Is OnetoOne Determine whether the following functions are onetoone (a) For the following function, the domain represents the age of five males and the range represents their HDL (good) cholesterol (b)The Collection contains problems given at Math 151 Calculus I and Math 150 Calculus I With Review nal exams in the period 0009 The problems are sorted by topic and most of them are accompanied with hints or solutions The authors are thankful to

Polynomial Functions

Relations And Functions Video Lessons Examples And Solutions

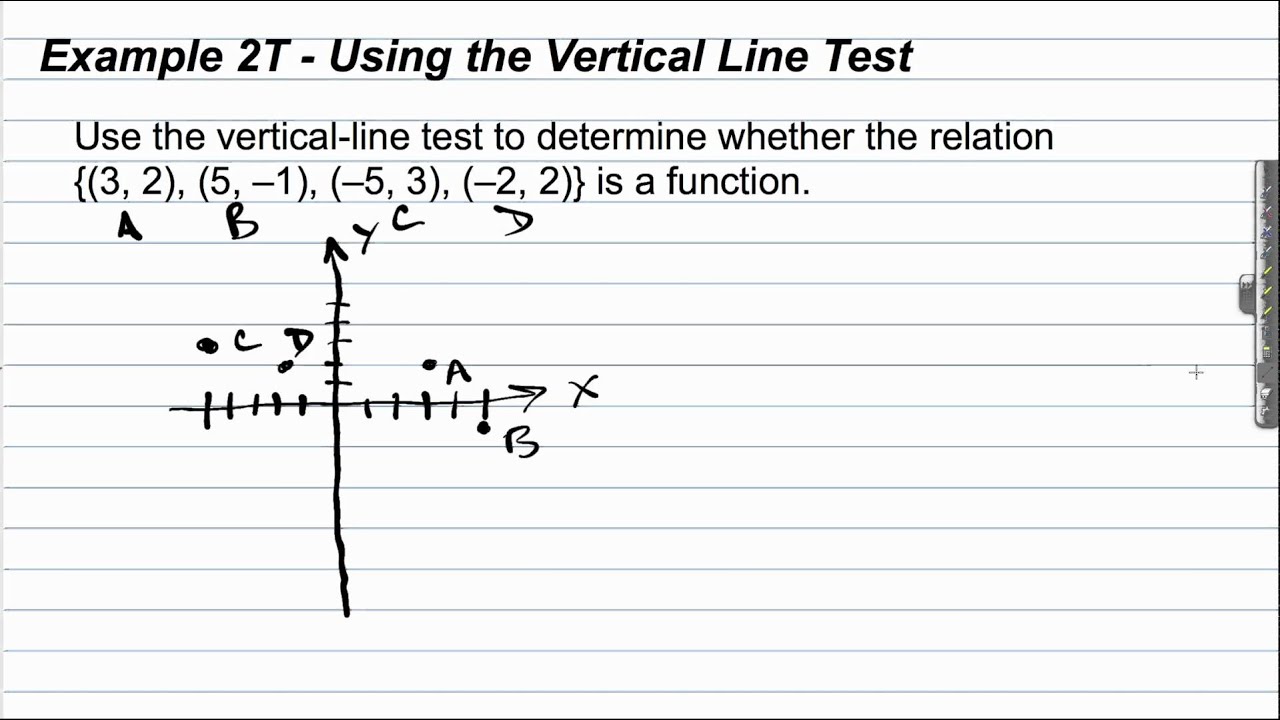
4 CHAPTER 1 NORMED AND INNER PRODUCT SPACES Solution We show that the norm kk1 does not satisfy the parallelogram law Let f(x) = 1 and g(x) = 2x Then kfk1 = Z 1 0 1dx = 1;Show that all functions of the form f (x) = a (x h) 2 k , for x >= h , where a, h and k are real numbers such that a not equal to zero, are one to one functions Solution to Question 5 We start with f (A) = f (B) a (A h) 2 k = a (B h) 2 k Add k to both sides of the equation to obtainExample 3 Solution For each, we only get one y, so this a function of Ans This is an implicit function of Note If we have a graph, we may determine if we have a function of by using the Vertical Line Test (if any vertical line hits the graph more than once, it is not a function of ) For example not a function of 17

Introduction To Piecewise Functions Algebra Video Khan Academy

Composite Functions Video Lessons Examples And Solutions

Example 3 Given the objective function C x y= 12 4 and the following feasible set, A Find the maximum value B Find the minimum value Solution Notice that the feasible set is unbounded This means that there may or may not be an optimal solution which results in a maximum or minimum function value The vertices (cornerThe lesson is that the class of unknown functions must be precisely prescribed If other curves are admitted into \competition" the problem may change For example the only solutions to minimizing Ly · def Z b a (1¡(y0) 2) dx;98 · For example, if the objective function is to maximize the present value of a project, and X i is the ith possible activity in the project, then c i (the objective function coefficient corresponding to X i ) gives the net present value generated by one unit of activity i As another example, if the problem is to minimize the cost of achieving

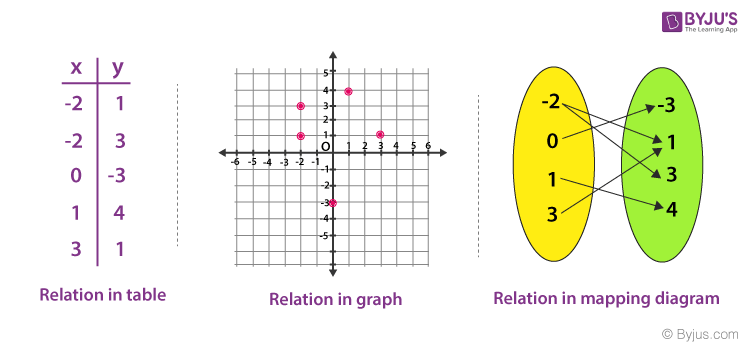
Relations And Functions Definition Types And Examples

How To Determine If A Function Is One To One Mathematics Stack Exchange

Have the following techniques to prove a function is onetoone (or not onetoone) • to show f is onetoone, take arbitrary x1,x2 ∈ X, suppose that f(x1) = f(x2) and try to deduce that this implies x1 = x2 • to show that f is not onetoone, find specific x1,x2 ∈ X with x1 6= x2 but f(x1) = f(x2) (ie provide a counterexample)Problem Let X and Y be jointly continuous random variables with joint PDF Show the range of (X, Y), RXY, in the x − y plane Find the constant c Find the marginal PDFs fX(x) and fY(y) Find P(Y < 2X2) Solution Figure 58 (a) shows R X Y in the x − y plane The figure shows (a) R X Y as well as (b) the integration region for finding P ( Y < 2 X 2) for Solved Problem 1Global Optimization Toolbox provides functions that search for global solutions to problems that contain multiple maxima or minima Toolbox solvers include surrogate, pattern search, genetic algorithm, particle swarm, simulated annealing, multistart, and global search

Function Definition Types Examples Facts Britannica

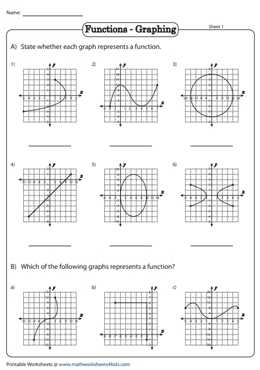
Identifying Functions Worksheets

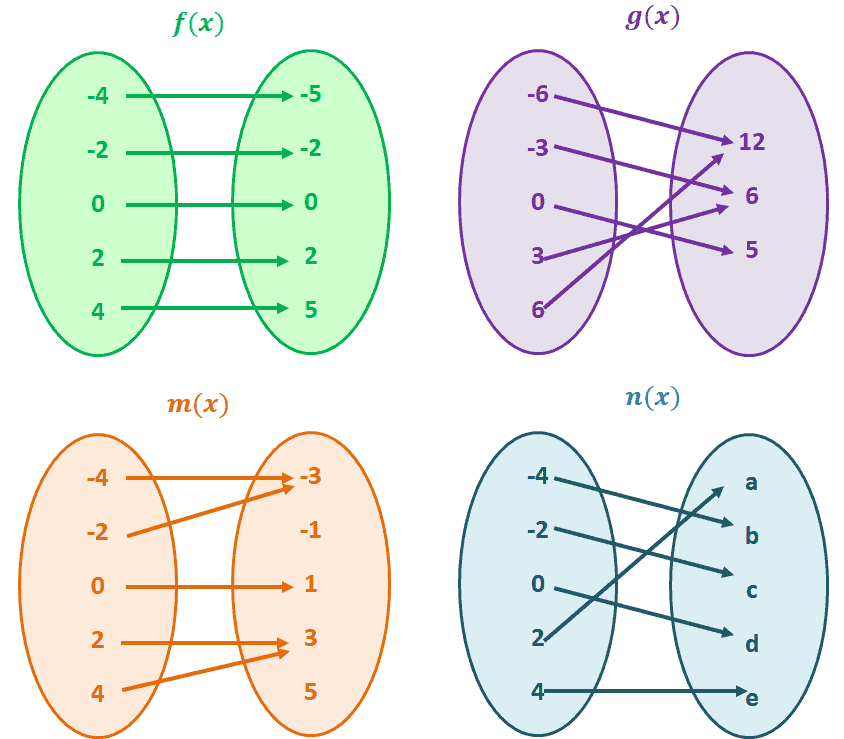
1 You are correct, if you set f(m) = f(n) then you can simplify that equation to n = m, and this is the definition of onetoone Conversely you have observed that f(n) is always odd This is because 2n is even therefore 2n − 1 is oddExamples – Now let's look at a few examples to help demonstrate what a one­ to ­one function is Example 1 Determine if the function f = {(7, 3), (8, –5), (–2, 11), (–6, 4)} is a one­to ­one functionMeaning of the quantities in the problem Looking at the table we see there are two solutions n = 2 and n = 4 When we input 2 into the function g, our output is Q Graph 1 is not a onetoone function For example, the output value 3 has two corresponding input values, 2 and 23

Composite Functions Video Lessons Examples And Solutions

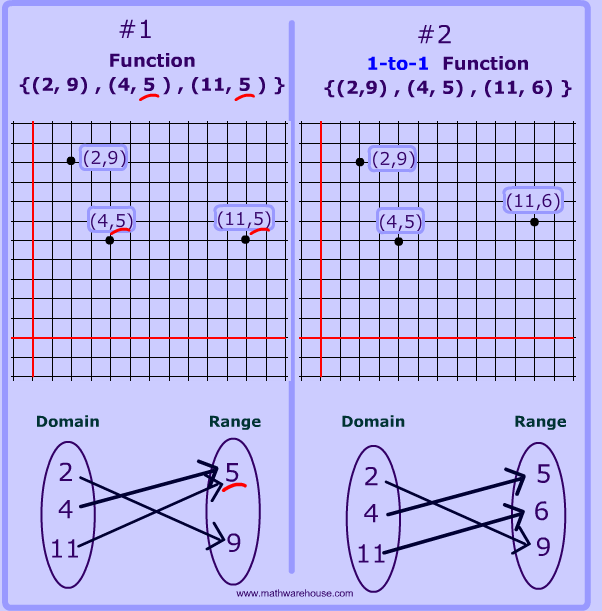
One To One Function Is The Inverse Of A Function A 1 To 1 Function Is Just

Word problems on average speed Word problems on sum of the angles of a triangle is 180 degree OTHER TOPICS Profit and loss shortcuts Percentage shortcuts Times table shortcuts Time, speed and distance shortcuts Ratio and proportion shortcuts Domain and range of rational functions Domain and range of rational functions with holes · NOT OnetoOne This absolute value function has yvalues that are paired with more than one xvalue, such as (4, 2) and (0, 2) This function is not onetoone In addition, values less than 0 on the yaxis are never used, making the function NOT onto Example 3 Is g (x) = x – 2 onetoone where g R→0,∞) With set B redefined toClick HERE to return to the list of problems SOLUTION 8 Evaluate It may not be obvious, but this problem can be viewed as a differentiation problem Recall that If , then , and letting it follows that Click HERE to return to the list of problems SOLUTION 9 Differentiate Apply the chain rule to both functions

Math Functions And Relations What Makes Them Different And How To Find The Domain And Range

Types Of Functions Classification One One Onto Videos And Examples

Onetoone function x 1 Z x 2;Thus the mapping must be onetoone M Hauskrecht Bijective functions Theorem Let f be a function from a set A to itself, where A is finite Then f is onetoone if and only if f is onto Please note the above is not true when A is an infinite set • Example –f Z Z, where f(z) = 2 * z – f is onetoone but not onto •1 2 •2 4Therefore, the solution to the problem ln(4x1)3 = is x ≈ Now that we have looked at a couple of examples of solving logarithmic equations containing terms without logarithms, let's list the steps for solving logarithmic equations containing terms without logarithms

Types Of Functions Javatpoint

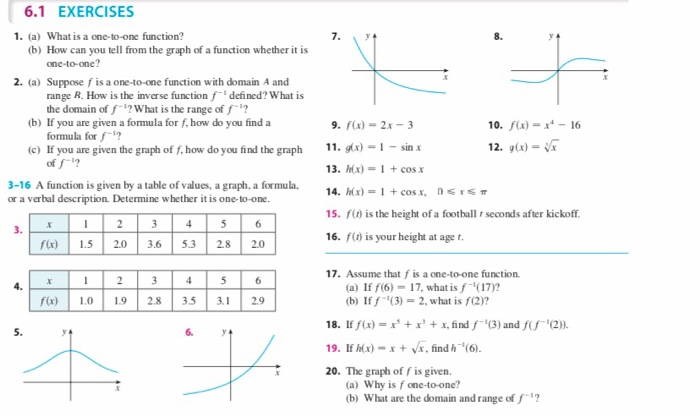
Solved 6 1 Exercises 7 1 A What Is A One To One Funct Chegg Com

Theorem 7 • If f0(x) > 0 for all x, then f is increasing, thus onetoone • If f0(x) < 0 for all x, then f is decreasing, thus onetoone Examples 8 • f(x) = x31 2 xisonetoone, since f0(x) = 3x2 1 2 > 0 for all x • f(x) = −x5−2x3−2xisonetoone, since f0(x) = −5x4 −6x2 −2 < 0 for all xUse a table to decide if a function has an inverse function Use the horizontal line test to determine if the inverse of a function is also a function Use the equation of a function to determine if it has an inverse function Restrict the domain of a function so that it has an inverse function Word Problems – Onetoone functionsAnd (2) to develop an intuition, and \feel" for

One To One Functions Mathbitsnotebook Ccss Math

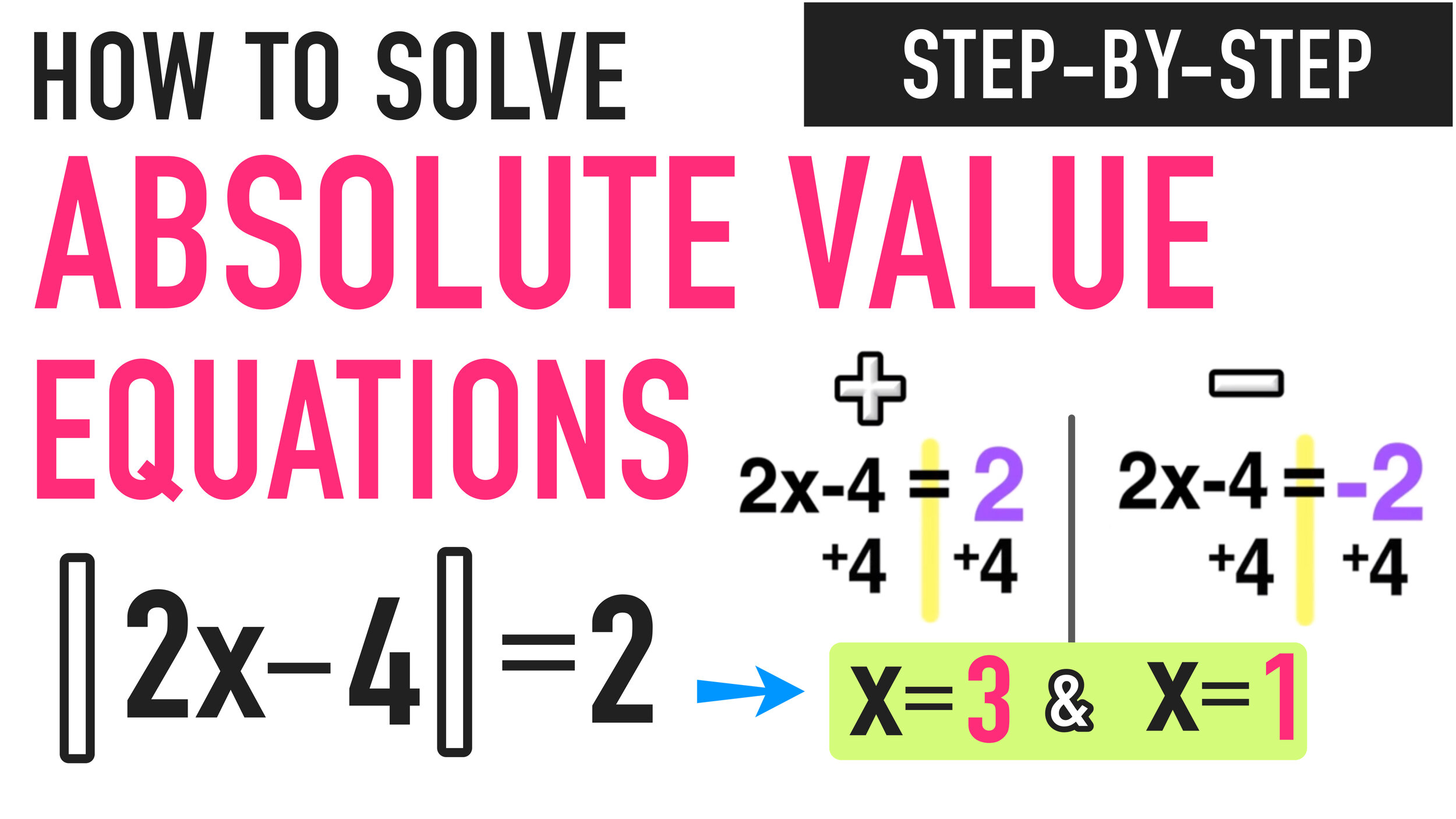
Solving Absolute Value Equations Complete Guide Mashup Math

 · Here is a set of practice problems to accompany the Functions Section of the Review chapter of the notes for Paul Dawkins Calculus I course at Lamar University Paul's Online Notes Practice Quick Nav Download@z @x = f0(x y) y Thus zyFunctions, composition of functions, images and inverse images of sets under functions, nite and in nite sets, countable and uncountable sets On the one hand all these are technically prerequisite to a careful discussion of the foundations of calculus On the other hand any attempt to do all this

One To One Function Explanation Examples

Injective Surjective And Bijective

Example 2 Show that the function f Z → Z given by f(n) = 2n1 is onetoone but not onto For functions from R to R, we can use the "horizontal line test" to see if a function is onetoone and/or onto The horizontal line y = b crosses the graph of y = f(x) at precisely the points where f(x) = b So f is onetoone if no horizontal line crosses the graph more than once, and onto if everyLinear Function Word Problems Exercise 1 Three pounds of squid can be purchased at the market for $18$ dollars Determine the equation and represent the function that defines the cost of squid based on weight Exercise 2 It has been observed that · For example, the quadratic function, f(x) = x 2, is not a one to one function Let's look at its graph shown below to see how the horizontal line test applies to such functions Let's look at its graph shown below to see how the horizontal line test applies to such functions

One To One Function Injective Function Definition Graph Examples

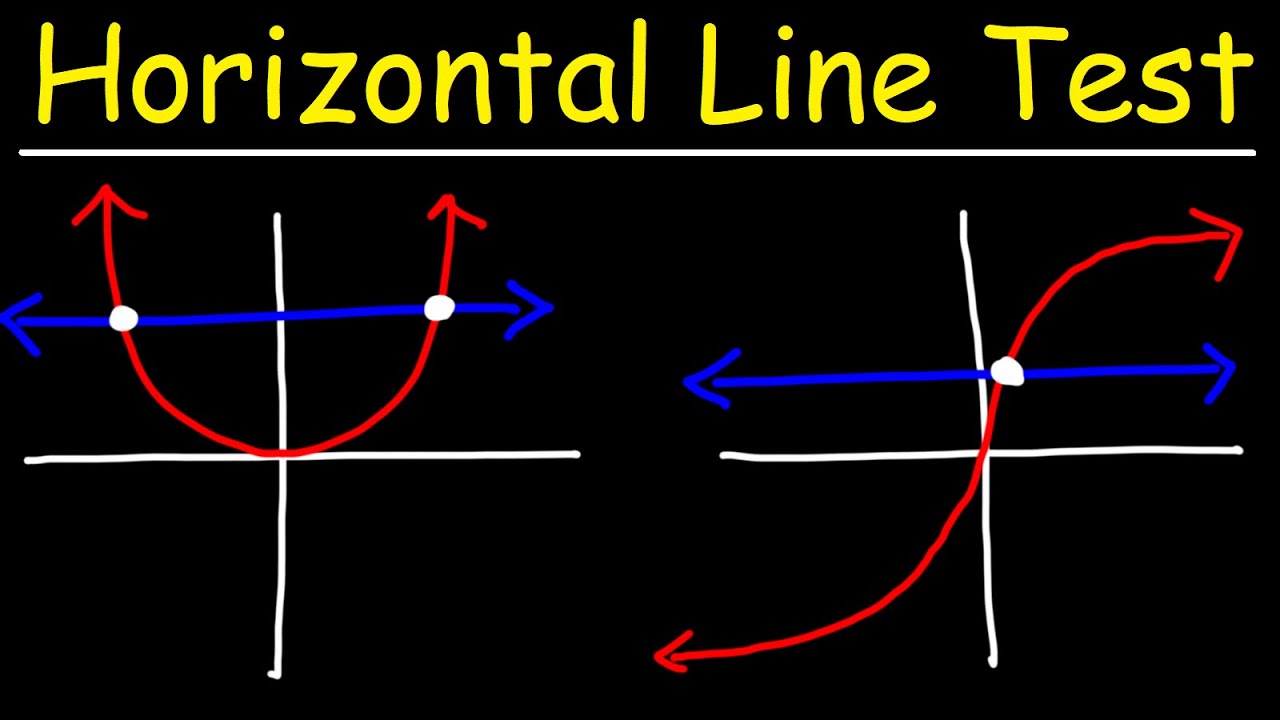
Horizontal Line Test And One To One Functions Youtube

The remaining 4 are defined in terms of them Example 11 Simplify the expression tanh ln x Solution EOS Note that we simplify the given hyperbolic expression by transforming it into an algebraic expression Go To Problems & Solutions Return To Top Of PageKf gk1 = Z 1 0 j12xjdx = 2 Thus, kf ¡gk2 1 kf gk 2 1 = 17 4 6= 2( kfk1 kgk2 1) = 4 ¥ Problem 3 Consider the linear space C0;1 equipped withOnetoOne Functions on Infinite Sets Now suppose f is a function defined on an infinite set X By definition, f is onetoone if, and only if, the following universal statement is true Thus, to prove f is onetoone, you will generally use the method of direct proof suppose x 1 and x 2 are elements of X such that f (x 1) = f (x 2) and show that x 1 = x 2

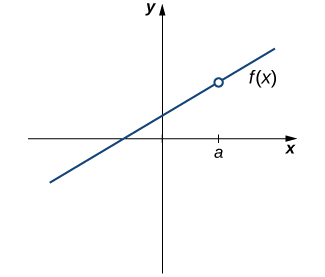
2 4 Continuity Calculus Volume 1

Discontinuous Functions

Print OnetoOne Functions Definitions and Examples Worksheet 1 While reading your textbook, you find a function that has two inputs that produce the same answerProblems and Solutions Exercises, Problems, and Solutions Section 1 Exercises, Problems, and Solutions Review Exercises 1 Transform (using the coordinate system provided below) the following functions accordingly Θ φ r X Z Y a from cartesian to spherical polar coordinates 3x y 4z = 12 b from cartesian to cylindrical coordinates y2 zSome Worked Problems on Inverse Trig Functions Simplify (without use of a calculator) the following expressions 1 arcsinsin(ˇ 8) 2 arccossin(ˇ 8) 3 cosarcsin(1 3) Solutions 1 Since arcsin is the inverse function of sine then arcsinsin(ˇ 8) = ˇ 8 2 If is the angle ˇ 8 then the sine of is the cosine of the complementary angle ˇ 2

Implicit Differentiation W Examples And Worksheets

How To Determine If A Function Is One To One Mathematics Stack Exchange

While kf ¡gk1 = Z 1 0 j1¡2xjdx = 1 2;Problem Set 7 Solutions ECON 301 Intermediate Microeconomics Prof Marek Weretka Problem 1 (Production Functions) (a) The isoquants for each of the three production functions are show below f(K;L) = K2L K L f 1 f 2 (f 1

Functions And Relations Functions Siyavula

Solved Determine If The Following Functions Are One To On Chegg Com

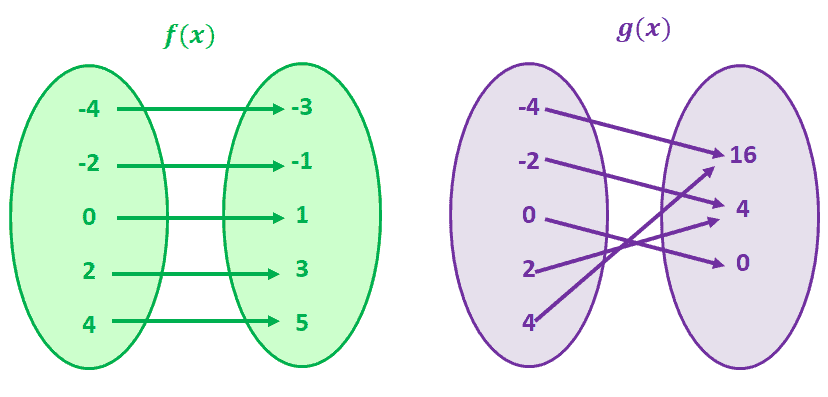
One To One Function Explanation Examples

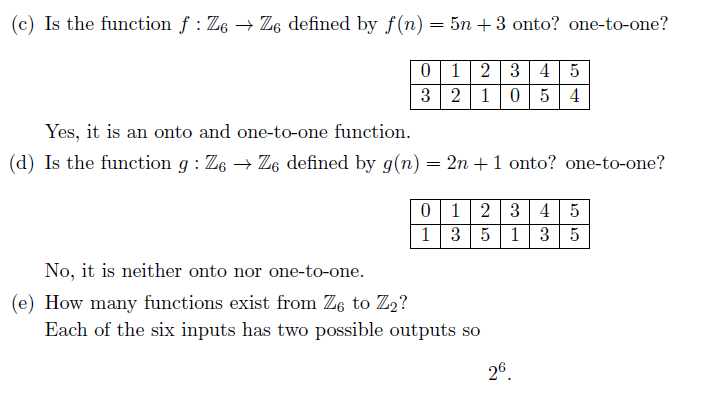
Solved Help With Discrete Mathematics Functions I Need He Chegg Com

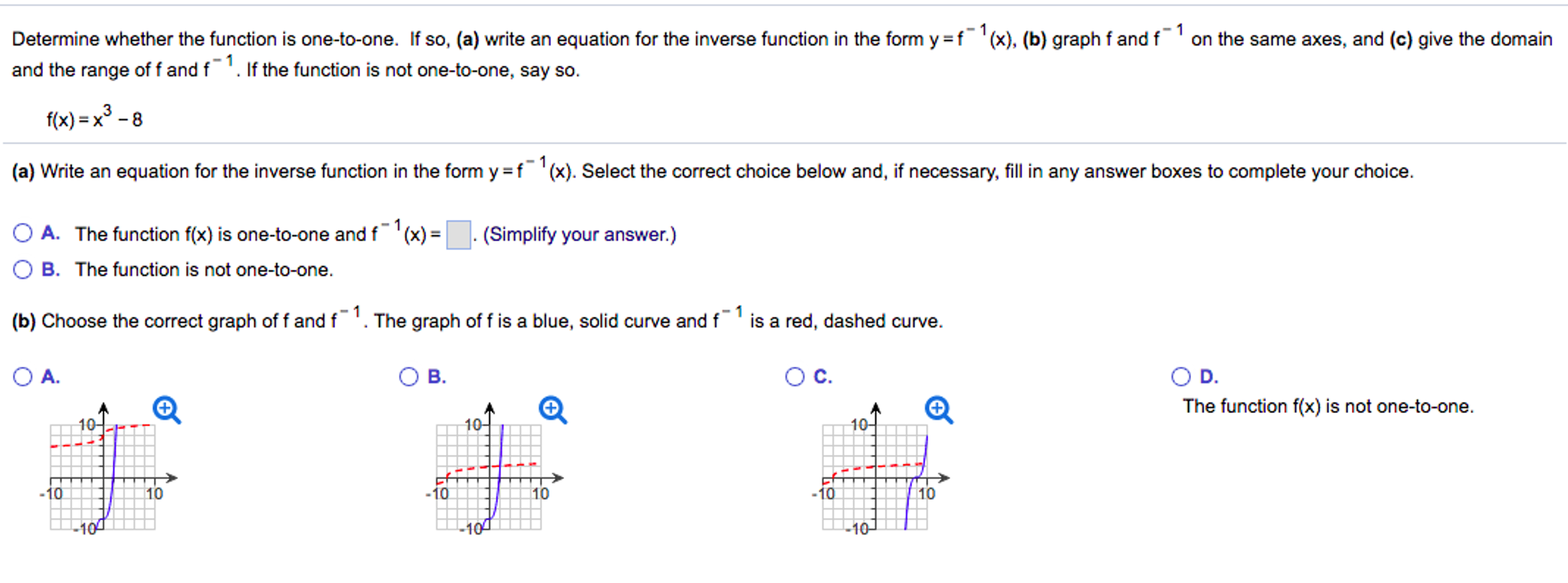
Solved Determine Whether The Function Is One To One If S Chegg Com

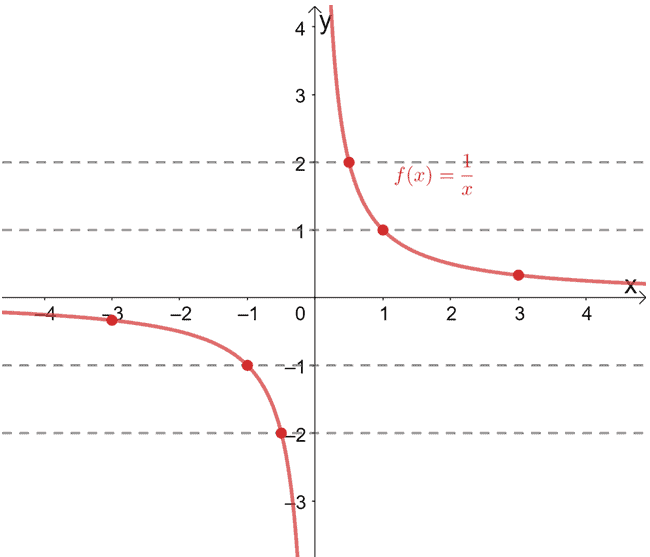
One To One Function Explanation Examples

Types Of Functions Classification One One Onto Videos And Examples

Relations And Functions Definition Types And Examples

Www Math Uh Edu Jiwenhe Math1432 Lectures Lecture01 Handout Pdf

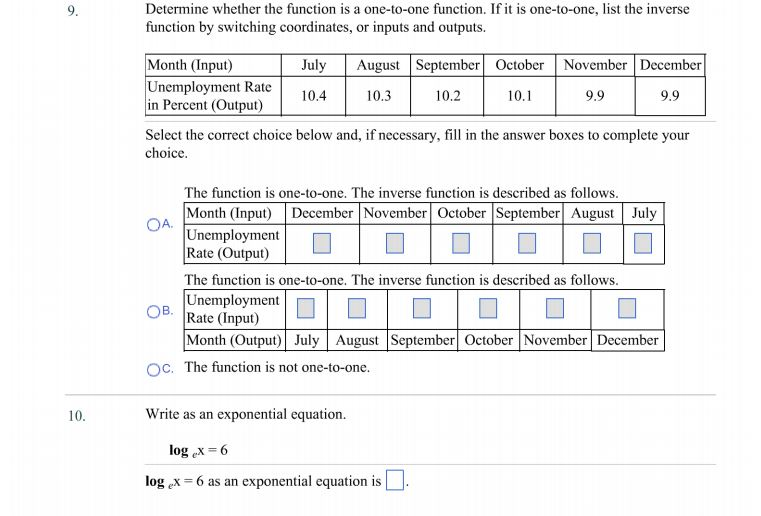
Solved Determine Whether The Function Is A One To One Fun Chegg Com

Determine If A Function Is One To One Youtube

Solved Determine Whether Each Function Is A One To One Fu Chegg Com

Types Of Functions Classification One One Onto Videos And Examples

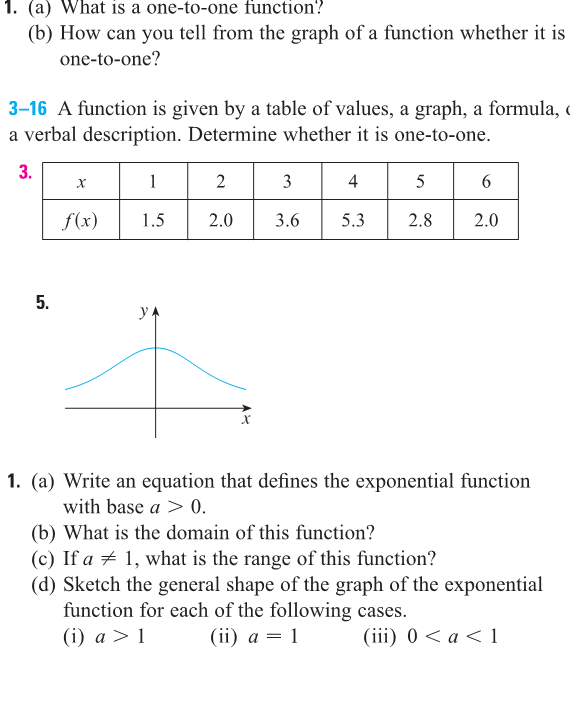
Solved What Is A One To One Function How Can You Tell Fr Chegg Com

1

Function Definition Types Examples Facts Britannica

One To One Function Is The Inverse Of A Function A 1 To 1 Function Is Just

Types Of Functions Javatpoint

Equation Word Problems Worksheets

College Algebra Chapter 4 Exponential And Logarithmic Functions Ppt Download

Math Functions And Relations What Makes Them Different And How To Find The Domain And Range

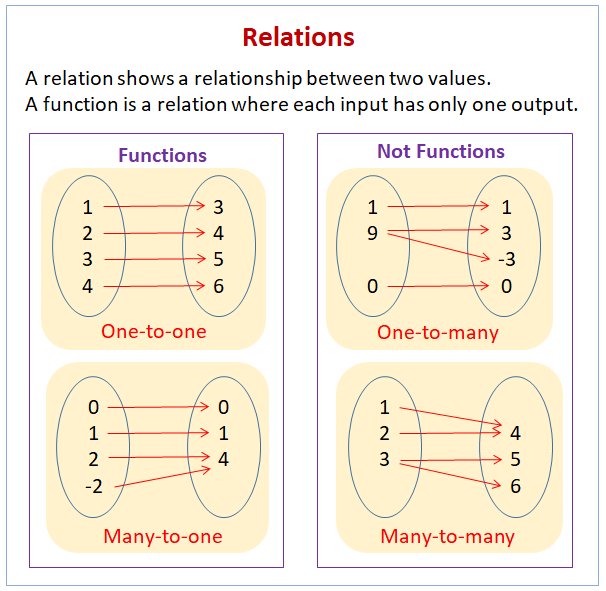
Relations And Functions Video Lessons Examples And Solutions

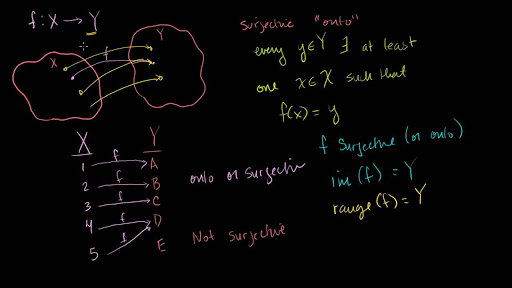
Surjective Onto And Injective One To One Functions Video Khan Academy

Solved For The Functions Below Show If The Function Is O Chegg Com

Identifying Functions Worksheets

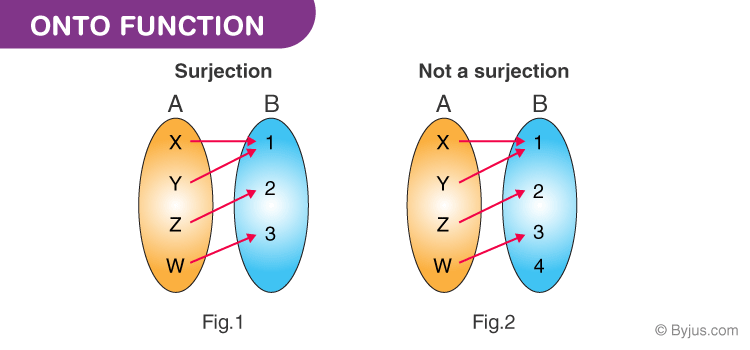
Onto Function Definition Formula Properties Surjective Function

Discontinuous Functions

One To One Functions Mathbitsnotebook Ccss Math

Bijection Wikipedia

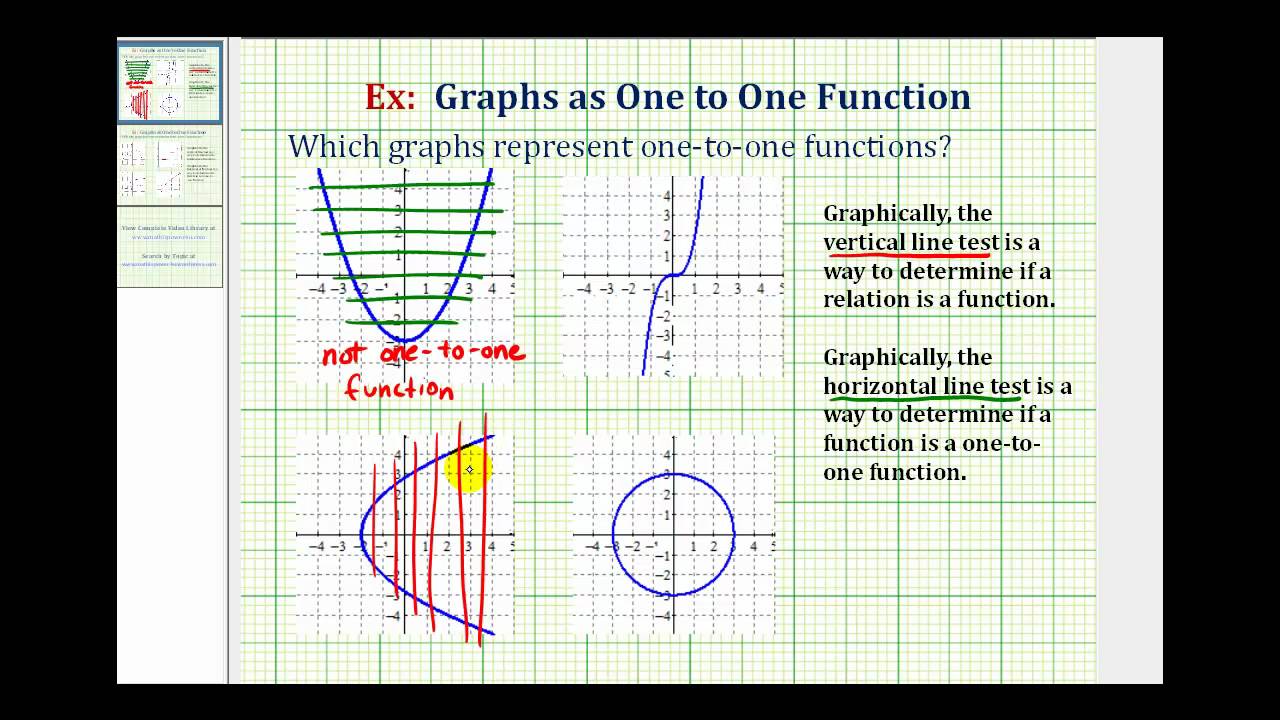
Use The Vertical Line Test To Identify Functions College Algebra

One To One Function Explanation Examples

One To One Functions Mathbitsnotebook Ccss Math

Free 2nd Grade Math Word Problem Worksheets Mashup Math

1

Identifying Functions Worksheets

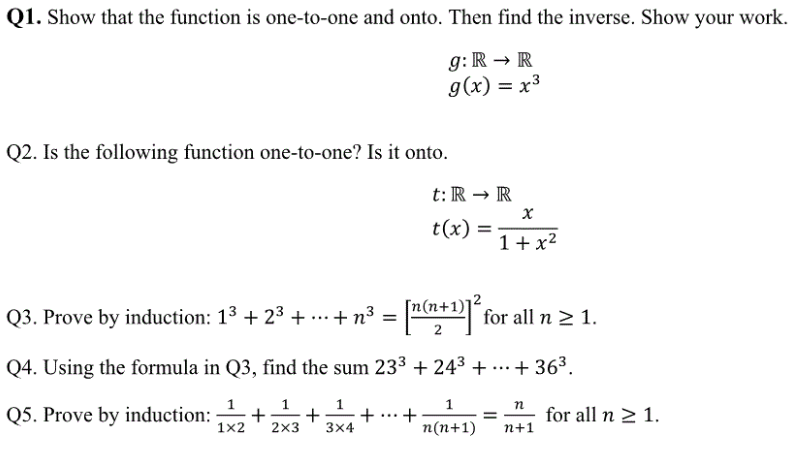
Solved Q1 Show That The Function Is One To One And Onto Chegg Com

2

One To One Function Injective Function Definition Graph Examples

1

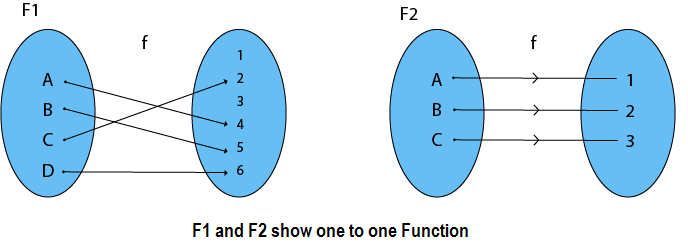
Types Of Functions Javatpoint

Pdf Section 2 7 One To One Functions And Their Inverses One To One Functions Arvin Squareroot Academia Edu

Ece 586bh Problem Set 6 Problems And Solutions Revenue

Applications Of Quadratic Functions Ck 12 Foundation

Solved Determine Whether Each Function In Exercises 10 15 Chegg Com

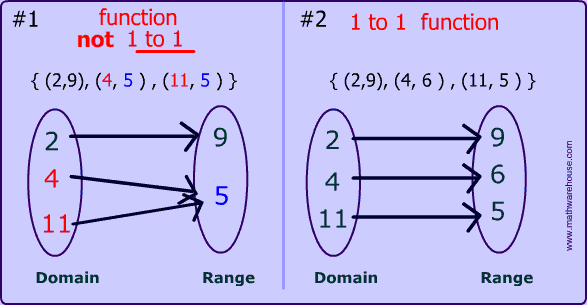
One To One Function Is The Inverse Of A Function A 1 To 1 Function Is Just

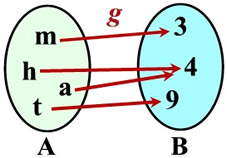
One To One Functions Mathbitsnotebook Ccss Math

Functions And Relations Functions Siyavula

Solved For Each Of The Functions Below State Whether F I Chegg Com

Grade 8 Unit 10 Homework Pdf Homework Problems Name Team Name Team Did Not Agree On Questions U26 Team Complete U19s Quick Look Write The Vocabulary Course Hero

Surjective Onto And Injective One To One Functions Video Khan Academy

Mathwords One To One Function

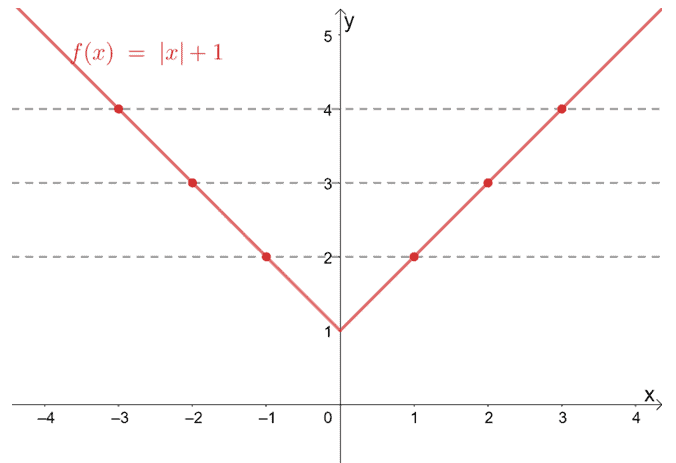
One To One Function Explanation Examples

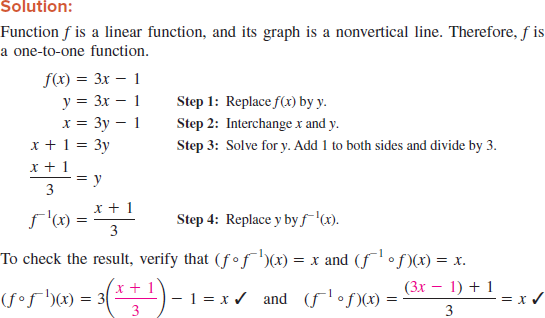
Solved For Exercise A One To One Function Is Given Write An Equ Chegg Com

Linear Programming Solution Examples

Copyright C Cengage Learning All Rights Reserved Ppt Download

Solving Quadratic Equations Using Tables Texas Gateway

Bijection Wikipedia

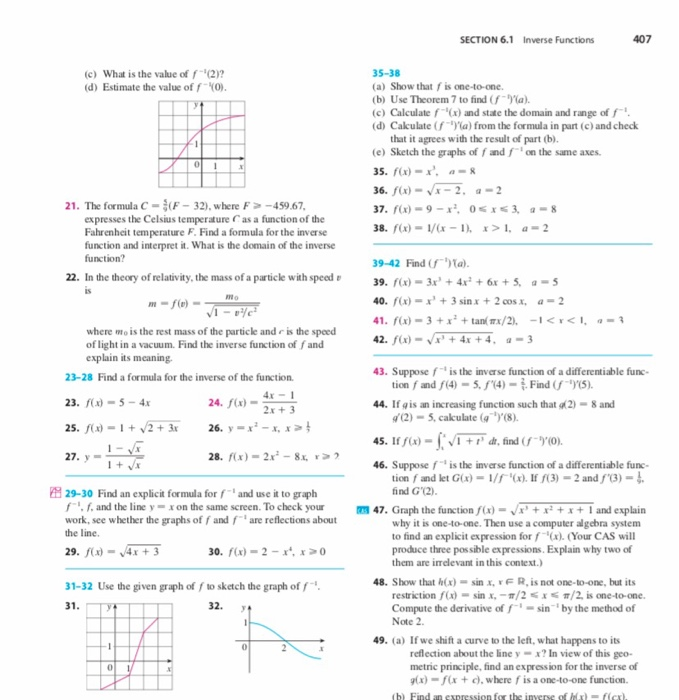
Solved 6 1 Exercises 7 1 A What Is A One To One Funct Chegg Com

5th Grade Math Word Problems Free Worksheets With Answers Mashup Math

Types Of Functions Classification One One Onto Videos And Examples

Types Of Functions Classification One One Onto Videos And Examples

Www Math Uh Edu Jiwenhe Math1432 Lectures Lecture01 Handout Pdf

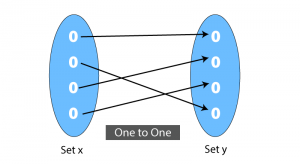
One To One Function Injective Function Definition Graph Examples

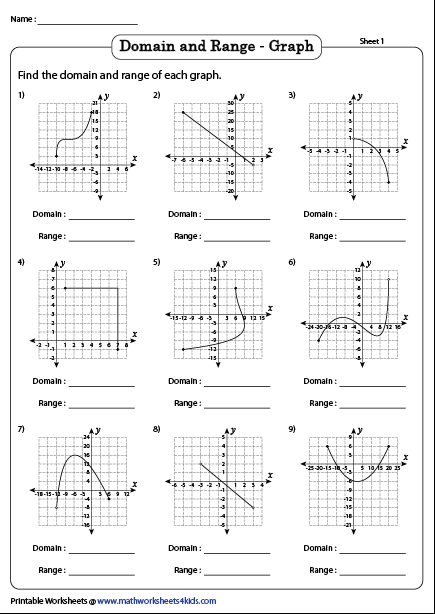
Function Worksheets

Types Of Functions Classification One One Onto Videos And Examples

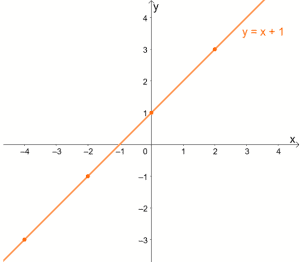
One To One Function Explanation Examples

Use The Vertical Line Test To Identify Functions College Algebra

Intro To Composing Functions Video Khan Academy

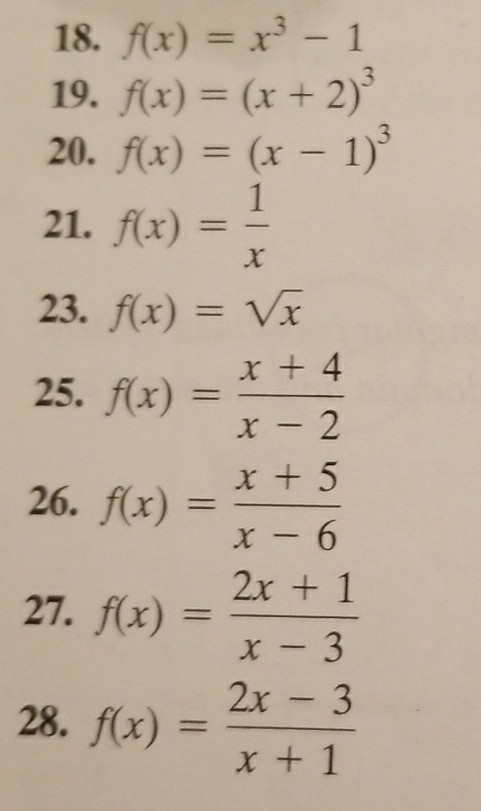
Solved The Functions In Exercises 11 28 Are All One To On Chegg Com

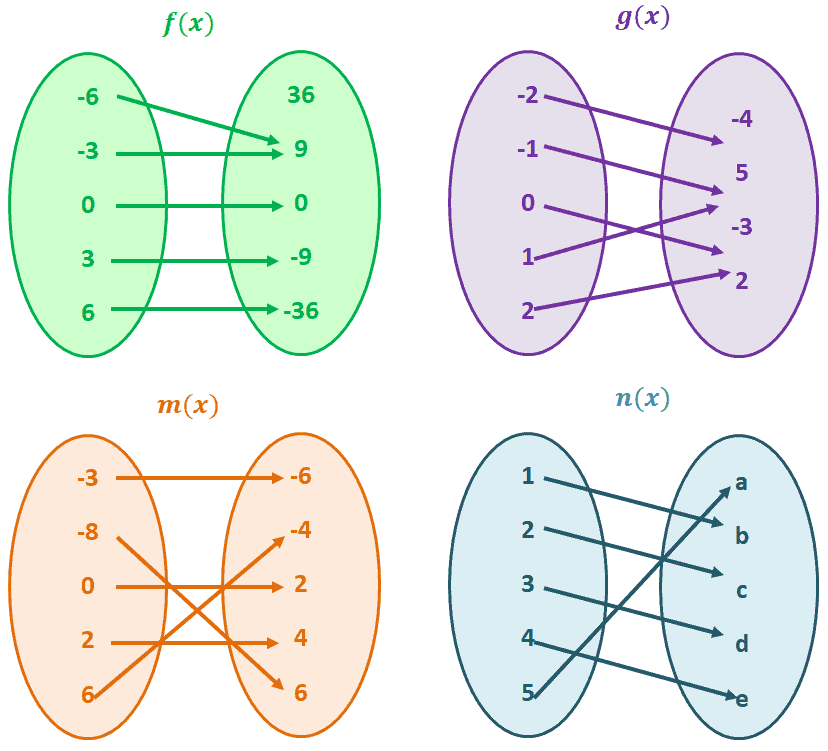
Mapping Diagrams

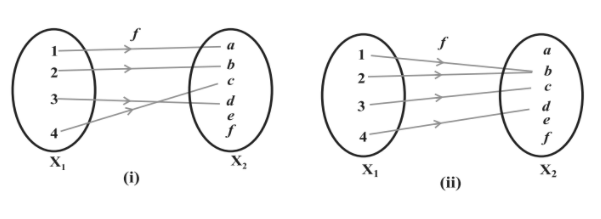
One To One Function Injective Function Definition Graph Examples

One To One Functions Mathbitsnotebook Ccss Math

Www Math Uh Edu Jiwenhe Math1432 Lectures Lecture01 Handout Pdf

Horizontal Line Test And One To One Functions Youtube

What Is The Horizontal Line Test Expii

1

Incoming Term: one to one function example problems with solutions pdf,